
Am Fam Physician. 2007;75(8):1231-1233
Clinical Question
What is the prognosis for a patient with acute exacerbation of heart failure?
Evidence Summary
Accurate risk stratification in patients with heart failure can help physicians choose the most appropriate place for hospitalization (general hospital ward or intensive care unit) and the intensity of care needed. Home management after initial stabilization in the emergency department may be appropriate for some low-risk patients.
A second study used a national registry of patients hospitalized with acute decompensated heart failure.2 A clinical decision tree (Figure 1) was developed using data from 33,046 hospitalizations between October 2001 and February 2003.2 The average age of patients was 72.5 years, about one half were women, and 53 percent had an ejection fraction less than 40 percent; the overall inhospital mortality rate was 4.1 percent.2
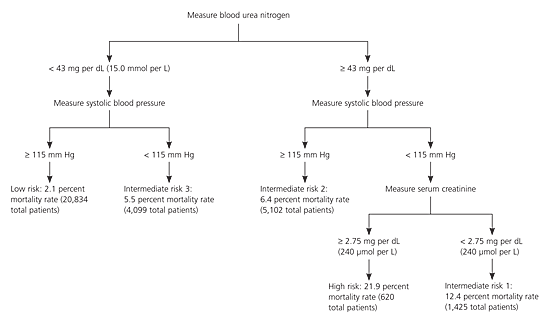
The best predictors of in-hospital mortality were identified using the classification and regression tree technique.2 This statistical technique includes testing the entire patient population to identify the best variable and cutoff value for distinguishing low- and high-risk patients, then similarly testing each subgroup until distinct risk groups are identified. The result is a clinical decision tree that is simple to apply at the point of care. The clinical decision tree was tested in a group of 32,229 patients hospitalized between March and July of 2003 and was found to be highly valid, identifying risk groups with in-hospital mortality rates between 2.3 and 19.8 percent.
A third study used data from 2,624 patients presenting with heart failure to Canadian hospitals between 1999 and 2001.3 A clinical decision rule (Table 13) was developed based on a logistic regression model and validated using data from 1,407 patients hospitalized between 1997 and 1999.3 The average age of patients in the validation group was 75 years, one half were women, and about one half had an ejection fraction less than 40 percent.3 The overall in-hospital mortality rate was 9 percent, the overall 30-day mortality rate was 11 percent, and the overall one-year mortality rate was 33 percent.3
| Clinical factors | Points | ||
|---|---|---|---|
| 30-day score | One-year score | ||
| Age (years) | + age in years | + age in years | |
| Systolic blood pressure (mm Hg) | |||
| < 90 | −30 | −20 | |
| 90 to 99 | −35 | −25 | |
| 100 to 119 | −40 | −30 | |
| 120 to 139 | −45 | −35 | |
| 140 to 159 | −50 | −40 | |
| 160 to 179 | −55 | −45 | |
| ≥ 180 | −60 | −50 | |
| Respiratory rate (breaths per minute)* | + rate in breaths per minute | + rate in breaths per minute | |
| BUN (mg per dL)† | + level in mg per dL | + level in mg per dL | |
| Serum sodium less than 136 mEq per L (136 mmol per L) | +10 | +10 | |
| Hemoglobin less than 10 g per dL (100 g per L) | +0 | +10 | |
| Patient history: | |||
| Cancer | +15 | +15 | |
| Cerebrovascular disease | +10 | +10 | |
| Chronic lung disease | +10 | +10 | |
| Dementia | +20 | +15 | |
| Hepatic cirrhosis | +25 | +35 | |
| Total: | ____ | ____ | |
Two risk scores are included in the clinical decision rule, one to predict 30-day risk and one to predict one-year risk. Thirty-day mortality ranged from 0.8 to 31 percent, whereas one-year mortality ranged from 9 to 66 percent.3
Applying the Evidence
A 72-year-old man is hospitalized with acute heart failure. His systolic blood pressure is 108 mm Hg, and his respiratory rate is 30 breaths per minute. The patient's blood urea nitrogen level is 44 mg per dL (16.0 mmol per L), his serum sodium concentration is 132 mEq per L (132 mmol per L), his serum creatinine level is 1.8 mg per dL (160 μmol per L), and his hemoglobin level is 11.2 g per dL (112 g per L). He has no history of cancer, cerebrovascular disease, chronic lung disease, dementia, or hepatic cirrhosis. What are his short- and long-term prognoses?
Answer: Based on the clinical decision tree (Figure 12 ), the patient is in the intermediate risk 1 group, with an in-hospital mortality rate of 12.4 percent. Using the other clinical decision rule (Table 13 ), he has a 30-day mortality risk of 30.5 percent (72 − 40 + 30 + 44 + 10 = 116 points) and a one-year mortality risk of 66.3 percent (72 − 30 + 30 + 44 + 10 = 126 points). You note that his scores are at the bottom of the very–high-risk range; therefore, his actual risk is probably somewhat lower, between the high-risk and very–high-risk groups.
